Zero is equal to unit

A zero is equal to unit - beautiful mathematical equality turns out. One variant of origin of similar equality did I consider in a note "Why is the factorial of zero equal to unit"? from the cycle of "Legend about mathematics". But this was just a joke on a theme a zero. Some time about this mathematical masterpiece will tell anecdotes. Statement that the factorial of zero is equal to unit it is possible bravely to attribute to the digit of mathematical funny things. But... let attentively we will look closely to equality a zero is equal to unit:

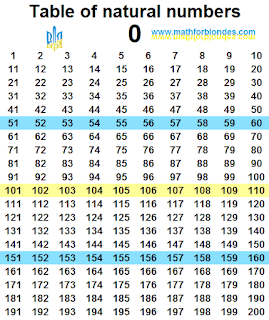
0 = 1

Looks beautifully. But there is a little problem in legitimacy of existence of similar equality - it we. Did you hear the fable of Ivan Krylov "Marmoset and glasses"?

Ivan Krylov. Marmoset and glasses.

This fable about us and about this equality. What will we do with this equality? Correctly, we will begin to stick this equality in all possible mathematical holes. Here simple example:

5 = 5 - undeniable equality, all marmosets approve amicably;

5 = 5 + 0 - was there obvious curiosity on the snouts of marmosets - now there will be something interesting, otherwise why to add a zero to the number?;

Presentiment of marmosets did not deceive - we use equality a "zero is equal to unit" and we put instead of zero unit:

5 = 5 + 1 - marmosets titter;

5 = 6 - marmosets amicably laugh at us, we in bewilderment.

"Any number is equal to any number" is the most terrible nightmare of mathematicians, which we so easily and simply got. Superstitious horror before similar equality is very well described in the story of Ted Chiang "Divizion by Zero". In this fantastic story woman-mathematician managed mathematical methods to divide a number into a zero. She got equality:

any number = any number

Finale of story "Divizion by Zero" tragic enough. But, here is a very interesting question: "Are there glasses by a that universal object which ideally goes Near ALL parts of body of marmoset?". We are not marmosets. We know a right answer - glasses are put exceptionally on a nose for the improvement of sight.

I would not begin to write about equality a "zero equal to unit", if this was the fruit of leisure fiction. But, strangely enough, it is the future of mathematics. That in what we today refuse to believe, tomorrow will be one of basic equalizations of mathematics. True, mathematics then will be other, not for monkeys with glasses.

Here little fact in support of my words. One of leading physicists-theorists, Andrei Linde, in the lecture "Many-sided Universe" said: "The sum of energy of matter and gravity energy in the scales of Universe is equal to a zero".

A zero can be equal to something, different from a zero! you will turn the special attention, that the question in this equality is about SUM, but not difference of two physical sizes. If physicists subtracted from one physical size other is be much simpler. As early as school we taught that if from something to subtract such is exact something, then as a result we will get a zero. But, physicists assure that we need to something to add something and as a result we will get a zero! The law of maintenance of energies in the scale of Universe equals a zero. Our equality a "zero is equal to unit" presents this law in a general view for any pieces, taken in the scales of universe. Strange, it turns out, what the amount of blondes in the scales of Universe must be equal to the zero... And it yet needs to be proved! Where do they, at that rate, go?!

Think, now to our marmosets not to the laughter. Does "this law of maintenance spread to all marmoset or only on her part? If on part, then on which one? If on all marmoset, then does this law operate on other marmosets? If does operate, then on all marmosets without an exception or only on select? If only on select, then who, at that rate, them does elect there? Write down and me!!! I want to be select!")))

As see, every new step in science generates mass of new questions. And for that, what not to look a marmoset with glasses, something needs to be understood. Though slightly.

How do you behave to mathematics?

"How do you behave to mathematics?" - such questioning I conducted in spring of 2010. In questioning 70 persons took part from a number the visitors of the Russian blog of "Mathematician for blondes". Here I bring the results of questioning and comments to them.

Most appeared those, who loves mathematics - 21 percent. It makes happy. But I do not want, what all of them grew into ordinary mathematical robots. I think, here physicists prevail on storage of thought in the hands of good teachers. Here can be lyric poets in the hands of talented teachers.

Consider mathematics interesting science are 11 percents. Mathematical slavery does not threaten this category of students. When them it will be compelled intensively to decide different family tasks and examples - they quickly will say to mathematics "no" and will go away from mathematics a little rather. I think, lyric poets which drove mathematicians with teachers prevail here.

Indifferently behave to mathematics are 14 percents. It is victims of ineffectual teachers. Teachers indifferently expound educational material and require the same indifferent answers in reply. For it the teachers of mathematics need to put large two.

Consider mathematics bad science are 15 percents. It is victims of terror from the side of ineffectual teachers. Ineffectual teachers consider that mathematics all must know. Lyric poets on such violence answer a hatred. Personally exact sciences were much easier given me. Languages and literature I hated. From all long-term school course of languages I memorized only one: a rule of grammar is this large black spot in a textbook, which needs to be learned. From a look to these rules became me depressed.

Those, whoever knows about what mathematics is 9 percents. As I suppose, these are blondes. To them I belong. I also try to understand, what such the mathematics. An existent set governed and determinations personally me does not arrange already. Too much I am questions, on which mathematicians simply unable to give an answer. Simplest example. Increasing length of two perpendicular parties of rectangle, we get meters square and we get an area. Increasing length of two parallel parties, we get meters square but we do not get the area of rectangle. Why? An answer "so can not be done" me does not arrange. An answer "so can not be done" me does not arrange. It is so "possible", and it is so "impossible" is already not mathematics, it is a spiritualistic session.

Wunderkindes and cretin with blondes

In comments to my report omeone said of the opinion: "It is the article from heading "Cretins write for blondes", probably. Correct, please". As an author of question felt free in expressions, I also will call a spade a spade and on me for it I ask not offended.

Really, normal such will not be written. At most, what the normal are capable on, so it is dull to teach someone once written. And than better they it is quoted then, the are more clever considered. Both religion and science sticks to thereon. Dull untalented mediocrity which considers itself a norm and which is managed by more sharp untalented to mediocrity turns out in the total. Any bureaucratic vehicle consists of them, from normal. All, who though by something from a norm differs, are considered fools and fools. What, I agree to be a fool or cretin which writes for fools and blondes.

I would not begin to watch out for this comment, if he was not the object-lesson of other problem. One scientific site on which solid scientists communicate and one of them formulated such question was here remembered me: "MANY CLEVER COLLECTIONS of TASKS And GUYS WHICH DECIDE THEM. WHY THEN SO FEW DISCOVERIS"?. Farther a few quotations are for illustration of problem:

I was always surprised by one circumstance. When look tasks which are offered in our collections of tasks on physics and on mathematics for high school and institute of higher, on school olympiads, at entering university and so ддалее, then there is the impression, that they are counted on supermen. In any event, suppose the very high level of possession material. And some tasks in a mathematical magazine "Quantum" - it in general, to my mind, whole research, counted on the experienced specialist. Moreover, appears, there are guys (and I personally by a sign not with one!) which all these the tasks decide easily.

Certainly, we have very clever and capable young people. Why then, if do we decide intricate problems so easily, we so few accomplish discoveris?

... By the way, the same phenomenon I look after in the West. Take in hands collection of tasks on physics for the graduate students of Massachusetts Institute of Technology or collection of tasks on a gravitation and theory of relativity edited from Saul Teukolsky. They on someone are counted. Them someone decides. But where is discoveris equivalent to the tasks, published in books?

It is a problem, lying inplane division of people on fools and normal. First let us understand with the decision of tasks. What task? It by someone the made set of basic data and question on which it is needed to give an answer. Problem definition supposes application already of the known method of decision. For the decision of task it is enough to learn material and apply the got knowledge. An ordinary calculator turns out. What quicker such calculator decides tasks, the he is considered more clever. There are even wunderkindes which tasks decide - as nuts break. All this system of tasks and decisions differs small what from the system of training of animals. For a wunderkind-calculator, instead of command, it is enough to formulate a task, id est to say what where it is needed to find.

All of it results in that at taught standard character of thought is produced. Standard tasks decide standard methods easily and simply. It is needed only to deviate from standards - problems begin here. Let us try to decide a task, which it is scientifically well-proven for, that this task can not be decided. Who will engage in the decision of such task? Only fools. Normal, and the more so clever, never such task to decide will not become. They know an answer, by someone once written: "Task does not have a decision". Will fools be able to decide this task? Improbably, because and clever, and fools use identical standard principles of decision. Who can decide a similar task? That, whoever knows that this task does not have a decision and whoever uses standard principles of decision. Quite naturally, what a decision will be acknowledged as a discoveri.

Here historical fact. When the American mathematician George Dantzig was the student of university, then was once late to a lesson and counted up the equalizations written on a board a homework. Equalizations seemed to him more difficult, than set usually, but in a few days he did a homework however. Appeared, that these were tasks on statistics, on the decision of that many scientists worked and that at that time were considered "undecided".

And now we will go back to fools-blondes. All consider them fools because:

at first, their character of thought differs from standard;
secondly, they memorize standards badly.

Based on aforesaid, I have all grounds to assert that one blonde has many more chances to do a discoveri, what at all wunderkind-calculators, together taken. You will memorize: among normal there are not genii.

Why is the factorial of zero equal to unit?

A factorial appeared in mathematics near 1800. In mathematics a factorial is name work of all natural numbers, including indicated. Designate a factorial an exclamation mark, written after a number.

5! = 1 х 2 х 3 х 4 х 5 = 120

The official version of appearance of factorial in mathematics I do not want to search, because I know perfectly, how it was actually. And there was all so.

Completing all letups on a theory and practice of factorial calculations, Scientist bore the creation on the court of Saint Mathematical Inquisition. Functions of supervision after mathematicians on behalf of Saint Mathematical Inquisition in this locality executed Saint Scientific Infirmities. Responsibility on him lay enormous, work was very much, more precisely, to do it was in general nothing. Therefore Saint Scientific Infirmities selflessly dug up in a nose. After this employment he was found by Scientist.

Executed in due form bureaucratic art, a folder with the theory of factorials lay down on feet before the eyes of Saint Scientific Infirmities. Infirmities were taken out by a finger from a nose, fastidiously made a wry face and began the same finger to leaf a folder. He checked content of folder for accordance to "Law on registration of papers, presented to Saint Mathematical Inquisition". Obvious occasion to say no to Scientist in consideration of his papers was not. To enormous regret of Saint Scientific Infirmities. Vexation affected his official. An at this time leading hand reached the form of Official Statement, Book of Registration of Visitors, Book of Registration of Incoming Documents, Book of Registration of Get-away Visitors and other treasures of Responsible Leading already.

Carefully collating everything, that was written by Scientist, with the "Explanatory dictionary for muddle-headed Bureaucrats. Rules of writing of Words and Letters" (this masterpiece was hidden from the eyes of visitors under a vulture "For the official use"), said Saint Scientific Infirmities:

"Your materials will be considered in the term set by Law".

His leading finger again submerged in a nose, continuing the interrupted work. It meant the end of audience.

The time of consideration of document fixed by law passed. A scientist again came to Saint Scientific Infirmities. Discontentedly made a wry face infirmity, scratched the back of head, feeding speech goes to remember about what, then got a folder with a factorial and began attentively it to study. An answer he must give today because the time taken on bureaucratic procedure made off already. Twisted infirmities backward, checking, firmly how enough Leading Arm-chair sticks to under them. Leading Arm-chair squeaked treacherously.

"You, Scientist, must know that a zero is a natural number", - said Infirmities and with relief breathed, - "All your factorials will equal a zero. On determination."

I want to remind readers, that events took place on a wild west, where a zero all consider a natural number.

"Your work very interesting. It will be really it is sorry me, if she will remain known to nobody", - continued Infirmities - "I would assist to the publication of your work, if you will add me in coauthors."

It is a widespread in science reception. Through him hacks do to itself a career in science. Suggestion of Infirmity did not surprise Scientist. He answered:

"I will be happy to be the coauthor of such prominent scientist, as you. But how to be with a zero?"

"I see no problems," - Infirmities demonstrated a complacency and grandeur, - "It is said In Saint Mathematical Limning, that any number, increased on a zero, equals a zero. But in this Limning there is not a single word about the factorial of zero. I will write an address to Saint Mathematical Inquisition with a request making alteration in text of Saint Mathematical Limning. Let them write, that the factorial of zero is equal to unit."

An agreement was attained. A scientist here entered a coauthor in the work. Wrote saint Scientific Infirmities appeal. This advanced study was sent for consideration of higher scientific leaders.

Higher scientific leaders knew the rules of bureaucratic games well. The name of Scientist they did not touch. The name of the inferior every higher scientific leader wiped and inscribed the name into place of coauthor. As a result of it a hole appeared in the coauthors of Scientist.

From the same pores in Saint Mathematical Limning there is a masterpiece of scientific thought: Gospel says of from Rules of Increase, that a zero, increased on unit, will be equal to the zero

0 х 1 = 0

A Gospel asserts from Factorial, that a zero, increased on unit, is equal to unit

0 х 1 = 1

Whew mathematics grows into a marasmus.

Why is trigonometry needed?

If to judge on that trigonometry which me unsuccessfully tried to teach at school and in the subsequent years of my studies, then trigonometry is thought of exceptionally in an order to complicate life to us. Sometimes, here and there, very rarely in life it was necessary to run into trigonometry, and that only because at school I was taught to decide some tasks through trigonometric functions.

Today I a few othergates I look at all things in general, and on trigonometry in particular. I became a blonde on character of thought and I see everything in characters, but not I learn by heart bluntly someone the thought of rules and determinations. So, from the height of flight of blond idea, I can bravely declare, whatever mathematicians understand, what trigonometry. They thought of many determinations, swept everything, that can be swept, in one heap, drew many pictures, made many tables glad, as children - present all of it to us. And we rush about with all this trigonometry, as a crackpot.

Why is trigonometry needed?

And meantime, trigonometry - it one of the most important things in the surrounding us world. It is needed only to dissociate trigonometry from other mathematical concepts which in trigonometry are ordinary garbage. you did culinary recipes read? We will "take a soupspoon that, we will add полстакана of it, will season here by this nasty thing and all is careful we will mix - a dish is ready, I ask to the table". But it is possible to prepare and on other principle: "take all edible, that will manage to find in a house, pour it in a large tub, carefully mix and gorge on a health, enjoy your meal". School trigonometry is prepared exactly on such recipe. Once I was handsomely tangled at the decision of geodesic task with the use of mathematical reference book. But it is other history.

I will make an effort, besides standard school letups, to show the component elements of different mathematical dishes to you. Skilful cooks use the comparatively limited set of foods, but can create all variety of national kitchens of the world. It is just possible to act and in mathematics. You will be very surprised, knowing, from what a few of component elements it is possible to create the most different mathematical masterpieces. The determination of natural numbers, integers and the brandname recipe of receipt of the greatest in the world number I already gave you. Let us a bit understand with school trigonometry, and then I will show you, as through trigonometry it is possible to measure one of most important for us things - is love.

Legend about Sine and Cosine (completion)

Legend about Sine and Cosine. Сompletion.

Beginning of Legend about Sine and Cosine

In decision of Mathematics, Sine and Cosine appeared forever bound in a direct corner. Exactly from a that mournful day, Day of Sentence, Sine and Cosine live on different parties of one direct corner. Unit untiringly watches after execution of sentence. Where was not one of brothers-twins, the second always will be in the distance, equal to Unit. If Sine will consider itself equal to Unit, Cosine grows into a zero. If Cosine becomes equal to Unit, Sine grows into a zero. Such is Sentence of Mathematics. In the different worlds he is named variously, Gods of him call "Sorrow of Sentence". In your world him it is accepted to name "Theorem of Pythagoras".

From the same pores Sine and Cosine never met and will no longer meet. There will not be more disputes between them about that, who of them main. Mathematics deprived them even rights to communicate inter se. As a mediator at the relations of Sine and Cosine Reverse Symmetry was appointed - for the unsurpassed talent of diplomat. When she socializes with Sine, then appears Tangent. She assures Sine, that he is more main, as is in a numerator, and Cosine - in the denominator of shot. When Reverse Symmetry socializes with Cosine, she sets up for Cotangent and assures Cosine, that he is more main, as takes seat in a numerator, and somewhere there, in to the bottom, in a denominator, there is Sine. After such socializing with Reverse Symmetry both Sine and Cosine feel incredibly happy, in fact the cherished dream of each came true of them - to be main.

For socializing with the surrounding world Sine and Cosine have a single circle. Any persons interested can pass from this circle either to Cosine or to Sine. They are always very glad to the guests and certainly the Sacred Books show to them, each it. Yes, exactly that book which it is written in, that he - main. With care wrapped in shreds, these books are kept each of them, as the most expensive relicts.

But rarely who of Gods dare glance in dwelling of Sine or Cosine. Gods know how jealously these two mites watch after guests, especially after those guests which go not to them. A guest failed to appear in what place of single circle, Sine knows exactly, what distance a guest will pass to Cosine. Cosine knows just, what distance dissociates a guest from Sine. Therefore swingeing majority of guests straight from a single circle ask interesting them distance and disappear on the businesses.

Fuss of single circle is almost continuous. Here always many visitors, not giving to miss to Sine and Cosine. But are in their life such minutes, on which even Gods try not to look...

In rare periods of calm, when the last visitor disappears from a single circle, Sine and Cosine go out to the corner in forty five degrees, again becoming indistinguishable. They turn the little persons to each other, trying to look over though something through endless distance of Unit... Almost not visible tears flow down On their cheeks... A Sine and Cosine with an inexpressible melancholy remember that distant and happy time. Time, when they were together...

Legend about Sine and Cosine (continuation)

Legend about Sine and Cosine. Сontinuation.

Beginning of Legend about Sine and Cosine

An anxious new that Sine and Cosine go to Mathematics, flew around the whole worlds quickly. Gods were in confusion. To this case they worked out all nascent problems. But now a problem arose up in the world of Numbers, and Numbers are not subject to Gods. Only Mathematics can establish order among Numbers.

Nobody of Gods never saw Mathematics all at once. Nobody knew exactly, that in their ideas about Mathematics is reality, and that by the figment of the imagination. All Gods sooner or later tried to know at Numbers, how correctly they understand one or another moment in the mathematical reasoning. In reply to all questions of Number enigmatically smiled and always pronounced a the same phrase: time will "Come, you will answer the question". Really, time passed and all became into the places. Some deductions were confirmed by life, some was dispersed, as fog. During all life of Gods their idea about Mathematics changed constantly.

And Gods got an exceptional case face to face to see Mathematics. She does not can to say no in attention to the faithful servants - to Sine and Cosine. Gods have a right to be present as the interested observers. From the decision of Mathematics will depend, in what world to live Gods farther.

When Sine and Cosine appeared before Mathematics, all looks of Gods were tied down only to Her. But Gods were waited by great disappointment - each of them saw that character which was by it created only. To not the least new detail.

What was seen to Mathematician by Sine and Cosine? Will pass many to time, before someone from Gods, possibly, will answer this question.

"That did bring you over to me?" - Mathematics asked at Sine and Cosine.

"We want, that you said, who of us main," - they pronounced.

"There are not main in family of Numbers. All Numbers are equal," - Mathematics repeated all the known truth.

"It is but as Unit?!" - Sine and Cosine exclaimed amicably.

"It is Unit such is exact Number, as all other numbers. Yes, at Unit the special status. But this status needs to be deserved. You know perfectly, to what Numbers and what this status is appropriated for," - Mathematics answered imperturbably.

"Then say to us, who is main from us two? We all the time argue and can not decide in any way," - stubbornly repeated over and over again Sine and Cosine.

"Reasonable creatures always can agree inter se. Unfortunately, you such are not," - Mathematics pronounced with bitter taste.

All Gods stopped beating in a fright. To declare reasonable creature unreasonable is means to deprive his rights on life. Did mathematics take away capital punishment to two representatives of immortal family of Numbers?! Such decision was not laid in consciousness of Gods. To give Numbers in power of Death is equipotent to capital punishment to the whole surrounding world!

"Do you want to be equal to unit? Do you want, that one of you always was more main than other? Do you want, that I stopped your dispute?" - Mathematics continued after short meditation. Anger was heard in voice of Mathematics, - "You are two capricious babies, which did not manage to become adults. Your place is in a corner," - Mathematics was slightly softened, Gods breathed with relief, - "Here my decision:"

sin²α + cos²α = 1

Gods stopped beating in bewilderment. They looked around on parties, trying to understand, what Mathematics did. Seemed, the world had remained former, but almost elusive differences were felt in everything. Gradually to Gods sense of this event began to come. Mathematics sentenced Sine and Cosine to perpendicular symmetry! Such usual to all World of Symmetry disappeared forever. The new world - World of Perpendicular Symmetry appeared.

To be continued.

A sine is 0 degrees, sin 0

The sine of zero of degrees equals a zero. On the picture of sine it looks so:

sin 0

Will you, certainly, ask: "And where, actually, sine on this picture"? And there is not he, he in a tiny hole from a zero hid. As a baby mouse in a burrow. To see a baby mouse, it is needed to trick out of him from a burrow. In the animated cartoons assert, the smell of cheese very helps on this business. Sine will not beckon cheese. But there is one piece which on a sine operates smoothly. This magic lure is named for sines is a corner. Not that which babies are put in, and that which in degrees or radians is measured. Let more attentive on we watch this hunt on sines.

We do not have a corner (a corner equals a zero) - there is not a sine.

sin 0° = sin 0π = 0

Now we will try to beckon a sine the littlest value of corner. We will look, as a sine will react on a corner in the zero of degrees, zero of minutes, one thousandth seconds:

sin (0° 0' 0,001") = 0,00000000484813681109

Do you see, the tag of curious spout appeared from a burrow? We will try to increase tenfold our lure and we will take a corner in one hundredth seconds.

sin (0° 0' 0,01") = 0,00000004848136811095

The amount of zeros before numbers grew short on one, and five appeared in the end. Certainly, in a burrow hidden yet very much цифер, which at a desire can be seen. It rather is a boa with a long tail from numbers. Yet tenfold we will increase a corner.

sin (0° 0' 0,1") = 0,00000048481368110954

Did you notice that numbers not nearly changed after zeros? It does not mean that a sine, as well as corner, increases exactly tenfold. Somewhere there, in distance from a comma, numbers change - a baby mouse moves a tail, but we it do not see. We watch only the first twenty numbers after a comma.

Here now for us unique possibility to admire the sine of one second in all his beauty (more precisely, his first two ten of numbers) appeared:

sin (0° 0' 1") = 0,00000484813681107637

We will look farther, as the first ten numbers change after a comma for 10, 20, 30, 40 and 50 seconds (quite naturally, that a superfluous tail we round off) :

sin (0° 0' 10") = 0,0000484814

sin (0° 0' 20") = 0,0000969627

sin (0° 0' 30") = 0,0001454441

sin (0° 0' 40") = 0,0001939255

sin (0° 0' 50") = 0,0002424068

It is possible to consider that for the sake of one minute a sine already fully abandons the burrow and begins quickly to hurry round us. You only will look at a sine 10, 20, 30, 40 and 50 minutes:

sin (0° 1') = 0,0002908882 

sin (0° 10') = 0,0029088780

sin (0° 20') = 0,0058177314

sin (0° 30') = 0,0087265355

sin (0° 40') = 0,0116352658

sin (0° 50') = 0,0145438977

I hope, you understand now, that when a corner arrives at all one degree, a sine becomes quite great. A little baby mouse grows into an adult mouse. Look, as quickly the sizes of sine change for corners in 1, 2, 3, 4, 5, 6, 7, 8, 9 and 10 degrees:

sin 1° = 0,017452

sin 2° = 0,034899

sin 3° = 0,052336

sin 4° = 0,069756

sin 5° = 0,087156

sin 6° = 0,104528

sin 7° = 0,121869

sin 8° = 0,139173

sin 9° = 0,156434

sin 10° = 0,173648

Some values of sine of corner alpha on the requests of visitors:

sin 17° = 0,292372

If you watching was not yet tired of a sine, then I suggest to pass to the page a sine 30 degrees. He can be not only seen there but also touch hands, at a desire.

That will be, if to increase a cosine on a sine?

In someone an inquisitive mind... Woke up If sine of corner alpha to increase on the cosine of corner alpha, a number, equal to the half of sine two alpha, will ensue. This statement hatches from the functions of multiple corners, where sine two alpha equals the doubled product of sine alpha on a cosine alpha. The picture of this trigonometric miracle I will show later:)

Sine is 45 degrees, sin 45

Sine 45 degrees, or sine, pi divided on 4 (four), equal unit, divided by a root from 2 (two). Simpler all sin to represent 45 degrees on a picture:

sin 45

In decimal fractions value of sine 45 degrees will be equal zero whole seven thousands seventy one tenthousandth:

sin 45° = sin π/4 = 0,7071

Anymore about trigonometric equalities of sine of corner alpha it is possible to look on a page, sanctified to the sine 30 degrees. I hope, you will understand, what numbers change in equalities, and what letters and symbols remain unchanging. You will compare the portraits of sines on pictures, value of corners and value of sines of these corners.

Now a bit searching phrases are for blondes, that they did not lose way in the searching systems. On this page you will find answers for such queries: sin 45 degrees, sin пи/4, what a sine is equal to 45 degrees, value of sines, value of sin, sin пи on 4, sin pi/4. Sine 45 degrees equal 1 divided by a root from 2. Value of sine, a sine what is equal to, how to find a sine, sine of acute angle - specially for blondes this page is created. Sine of number of pi on 4 radians, sine alpha of corner 45 degrees. Calculations of sine, even looking out a sine on a picture. Exhibit as a sine 45 degrees it is settled to touch hands. Before procedure to wash down being of sine of hand with soap.

Trigonometric sine - it just one of a few functions from family Functions Trigonometric. Where is pi divided by four? - in a district 45 degrees. Above there is a picture, it is drawn there, how to him to pass. Sine of pi divided by four - really, sexual belonging of number of pi somehow is not strongly paraded in mathematics. So nevertheless, pi - it he, she or it? The strangest, all answers correct. He is a corner of pi, she is pi, it is a number of pi. sin 45 what equal to? - to unit, divided by a root from two.

Devidend of fraction as named?

The devidend of fraction is named numerator. A numerator is always written above a fractional line. It is needed nowhere to peep in search of numerator of fraction, type under a bed. A numerator of fraction is always on the most prominent position, from above fractional line, as a prince on a pea. As a numerator there can be numbers or mathematical expressions.

How is a devidend named, that under a line?

In a fraction under a line a divizor is written. He is named denominator. If you must find the denominator of fraction, it is needed to search him under a fractional line. Type, how under a bed to glance. All denominators are always hidden under a fractional line, as under a bed. As a denominator there can be both numbers and whole mathematical expressions, sometimes very large.

Enter numbers the difference of numbers zero and twenty

"Enter numbers the difference of numbers 0 (zero) and 20 (twenty)" - a similar task very often meets in the Internet. Interesting that I do not know exactly, how her it is correct to decide. Omniscient Wikipedia reports bashfully, that a difference of numbers is a result of deduction of two numbers. But here, as correct to execute the action of deduction, this collection of wisdom holds back. In fact two variants of decision of this task are possible:

1. From the first number to subtract second 0 - 20 = - 20 (to take away zero twenty evened minus twenty)

2. From a greater number to subtract less 20 - 0 = 20 (twenty to take away zero evened twenty)

As see, we got two different answers. In one there is a sign minus, in other the sign minus it is not. Now we will begin to ratiocinate. A similar task is set by the program, the programs are written by programmers. I doubt that they remember about such nuances of school mathematics, as a sign is minus in the results of deduction of numbers. Therefore I suggest to operate by the tested method - by a scientific method. We will enter in a window numbers 20 (twenty) without a sign minus.

If Sim-Sim accepted our answer and opened access to treasures of the Internet, our experiment is completed. If a window answered us, that we are not right, then this iron will give out other pair of numbers to us.

Now we already the experienced users of this window. If it is again written us, for example, "Enter numbers the difference of numbers 0 (zero) and 16 (sixteen)", then we already know exactly, that it is needed in a window to enter - 16 (minus sixteen), with a mark minus ahead.

0 - 16 = -16

(zero minus sixteen evened minus sixteen)

If a window will write something type "Enter numbers the difference of numbers 1 (one) and 0 (zero)", then we without every vibrations enter a number 1 (one). In fact in this case and from the first number to take away second, and from a greater number to take away less, give an identical result is a positive number, without every signs minus.

1 - 0 = 1

(one minus zero evened one)

For all pair of numbers, if the first number anymore than second, a result will always be positive. For example:

20 - 14 = 6

(twenty minus fourteen evened six)

In a window it is needed to enter a number 6 (six, it a number is such).

In case if the program will take in head to flash the erudition and will give out to you a task "Enter numbers the difference of numbers 0 (zero) and 0 (zero)", not frightened and bravely enter a number 0 (zero)!

0 - 0 = 0

(zero minus zero evened zero)

Lighthouses for blondes. All, who searches answers for a question "That means enter the difference of numbers?" - you need to pass to this page!

Devidend of fraction as named?

The devidend of fraction is named numerator. A numerator is always written above a fractional line. It is needed nowhere to peep in search of numerator of fraction, type under a bed. A numerator of fraction is always on the most prominent position, from above fractional line, as a prince on a pea. As a numerator there can be numbers or mathematical expressions.

Greatest natural number

The greatest natural number is not present and can not be. Mathematicians at that rate it is said that the natural series of numbers are infinite. It is written so even in Wikipedia.

Let us understand, why takes place so. We will assume, we thought of the greatest natural number. For simplicity we will take the natural number of "Googol" is unit with one hundred zeros, ten in a hundredth degree.

Yes, yes, not surprised, the steepest searching machine of the Internet Google is named after the number of Googol! It and not surprisingly, in fact created a searching machine in distant, distant 1998 to the year two students Larry Page and Sergey Brin. You present, 12 (twelve!) years back there was not Google! How did people the Internet use?! But we were a bit distracted...

And so, we consider that the greatest natural number is a number of Googol. That us does interfere with to finish writing to this greatest number another, one hundred first, zero? We take a pen in hands, we look around on parties, that nobody saw, and we finish writing zero. Our greatest natural number increased tenfold and became yet more! Wow! We finish writing yet zero, and then yet, and yet... Through time, writing zeros already nowhere, and they (zeros) all do not end in any way. We reach the next piece of wallpapers, prepared for repair of antechamber, and we keep writing. On the middle of roll paste is closed, and the greatest natural number we so not wrote. If to buy up all ball-point pens in a booth and all wallpapers in a building shop, it how many zeros is it possible to finish writing? Will this be the greatest natural number? No, building магизинов with wallpapers very much, it is possible yet to write and write... Amusing, certainly, to spend right through life and all dad money on scribble of one number, but there are entertainments much more interesting.

Let now we will look at the problem of the greatest natural number on the other hand. If a child is able to consider only to five, then for such child pentad will be the greatest in the world number. But we know that well, that is yet very much many numbers which more pentad. Simply we know mathematics much better than child. In course of time a child will laugh at the "greatest in the world number".

There are no grounds to disbelieve mathematicians, to asserting, that the series of natural numbers are infinite and the greatest natural number can not be.

I tried to find the construction of the greatest number, but omniscient Wikipedia is quiet even on that score, and a search on the Internet is given out by different garbage. Therefore I present the own variant of the GREATEST NUMBER IN THE WORLD. It will look as endlessness in a degree endlessness, in a degree endlessness, in a degree endlessness... and so for ever and ever. Instead of mark of endlessness can put any natural number, except unit. What a greater number you will put, the steeper there will be flight to unattainable.

∞^{∞∞∞···∞···}

Here it and there is the GREATEST NUMBER IN MATHEMATICS, more precisely, his mathematical construction. If someone knows from erudites, how this is named, write in comments, with pleasure I will correct the name. Not forget to give reference to the information generator. Similar principle of search of the greatest number is much more effective than dull finishing writing of zeros.

By the way, little numbers at which small zeros grew quite have the famous enough names own. Glance on a page "Unit and twenty one zero", if want to become acquainted with them closer. Every blonde is under an obligation to know, what a millionaire differs from a multimillionaire. Otherwise as will you choose to itself a husband?

What number are the natural series of numbers begun with?

The natural series of numbers are begun with a number 0 (zero). Number 0 (zero) is the least natural number.

For the Russian blondes it was farther written following. In translating into the language of blondes: the littlest natural number - it 1 (one, unit). The littlest natural number has really two names. Probably, one from a dad, second from a mother))) I will remind that in Russian mathematics zero is not a natural number!

What natural numbers smaller five?

Among natural numbers there are five numbers which smaller 5 (five). It is numbers 0 (zero), 1 (one), 2 (two), 3 (three) and 4 (four). We will repeat for evidentness in large. Natural numbers smaller five:

0; 1; 2; 3; 4

Checking up the rightness of my reasoning is possible, overseeing in the table of natural numbers.

Legend about Sine and Cosine

I

Legend about Sine and Cosine. Вeginning.

n ancient, ancient times, when the world was quite another, and quite another laws governed in him, two nice tiny creatures, Sine and Cosine, went to school. They lived in long and friendly family of Numbers. Beat Sine and Cosine bytwins - nobody could distinguish them, even Lord of All Mathematician. Many different amusing histories happened with twins from their alikeness. They especially loved to jolly above Gods which built the worlds and often asked for a help at Numbers. Though quite tiny were Sine and Cosine as compared to other Numbers, but work was executed very important. One of twins will help to create a next universe God, God thanks Sine - and it, appears, Cosine tried in all...

Long proceeded so, or not, but once at school, sitting after one school desk, very strange phrases read Sine and Cosine. In the textbook of Sine it was written: "Sine is main, and Cosine helps him". In the textbook of Cosine it was written: "Cosine is main, and Sine helps him". In all other the textbooks were absolutely identical. Brothers were thoughtful right here, and then began to argue, who of them main. Each of them asserted that main he, and in his textbook it is written correctly.

Brothers argued long, finally, asked senior Numbers:

"Who of us main?"

Wise Numbers looked at textbooks, looked at brothers said:

"Change inter se textbooks - and all problems will disappear!"

But, neither Sine nor Cosine wanted to become helpers, they wanted to be only main! Everybody grasped the book and with renewed strength a dispute flamed up between them.

Then brothers appealed to Gods. Many reasonable creatures are known by Gods and with all able to get along with. They know how to settle a dispute.

"Who of us main? What book is it correctly written in?" - asked Sine and Cosine Gods. Gods looked on two absolutely identical tiny creations, smiled and said:

"It is not necessary blindly to believe in everything, that it is written in books. There can be a misprint in any book. Throw out these textbooks and live by the mind, it is time you to become adults."

Sine and Cosine got angry, hid the books a little rather from extraneous eyes. They became stealthily from each other to read each the book, more precisely, each the line about itself.

Long would proceed so, but once, on a call one of Gods, both brothers came for help. Instead of that to help God in his work, Sine and Cosine undertook a dispute inter se. God got angry and sent brothers to Mathematics.

To be continued.

Multiplication by zero

Multiplication by zero is possible, rules of mathematics multiplication by zero is not forbidden. Any number, multiplication by zero, will equal zero. If a whole or fractional number multiplication by zero, zero will ensue.

We will consider the example of multiplication by zero of integer. How many will it be, if 2 (two) to multiplication by 0 (zero)?

2 х 0 = 0

Decision: if 2 (two) to multiplication by 0 (zero), 0 (zero) will turn out.

Example of multiplication by zero of broken number. How many will it be, if 0,25 (zero whole twenty five hundredth) to multiplication by 0 (zero)?

0,25 х 0 = 0

Decision: if 0,25 (zero whole twenty five hundredth) to multiplication by 0 (zero), 0 (zero) will turn out.

If to multiplication a positive or negative number by zero, zero will turn out. A number zero does not have sign, therefore signs a plus or minus before zero is not put. Examples of multiplication of positive whole and fractional numbers are made a higher.

Example of multiplication by zero of negative number. How many will it be, if -2 (minus two) to multiplication by 0 (zero)?

-2 х 0 = 0

Decision: if -2 (minus two) to multiplication by zero, there will be 0 (zero).

Table division by zero

Division by zero is forbidden. Any number, positive or negative, whole or shot, to divide by zero is forbidden. Mathematicians say that the result of division by zero is undefined. Therefore a division table by zero will look so:

Table division by zero

If to designate any number through а, then a division table by zero for any numbers will consist only of one line (last line).

Why is the result of division by zero undefined? Because any number multiplied by zero equals zero. Since the mathematical operation of division is the inverse of the mathematical operation of multiplication, division by zero results in all the numbers at once. And mathematicians want to have one specific number.

Is it possible to solve the problem of division by zero? Yes, this problem can be solved. As a result of dividing by zero of a specific number, you will get another specific number. But this requires a much better understanding of mathematics.

I solved this problem. But at the same time, my views on some of the statements of mathematicians have changed dramatically. I spent a lot of time on this. Here you can compare your views on mathematics with the way I see it.

Division by zero

It is accepted to consider in mathematics, that division by zero not possibly, as a result of division of number by zero can not be certain. Yet mathematicians it is said that division of number by zero behaves to the mathematical operations, to not making sense. Wikipedia asserts on this occasion, that in arithmetic, division by a zero is forbidden. Therefore, when in examples there is division by zero, it is said that an example does not have a decision, as division by zero is forbidden. This mathematical rule behaves to all, even to the blondes.

It becomes firmly established in very clever mathematical books, that division by zero possibly. More precisely, mathematicians thought of sly tricks, what this division by zero to go round a side. They are sure that it succeeded them. So, if in conversation with a clever mathematician, you will hear a phrase "I am able to divide by zero!", not surprised, your interlocutor believes sincerely, that it is possible.

Sine is 30 degrees, sin 30

>Sine 30 degrees are evened by the one second or zero whole five tenth.

sin 30° = 1/2 or sin 30° = 0,5

In the radian measure sine of corners 30 degrees correspond a sine π/6:

sin 30° = sin π/6

Oddly enough, but justly and reverse equality, which asserts that sine π/6 (sin pi/6) equal a sine 30 degrees:

sin π/6 = sin 30°

A sine pi / 6 is evened similarly one second or zero whole five tenth.

sin π/6 = 1/2 or sin π/6 = 0,5

It was for blondes. For brunettes and bald academicians of mathematical sciences it is possible to write down all of it in a general view, let untangle:

sin 30° = sin π/6 = 1/2 = 0,5

For complete happiness here obviously the picture of sine 30 degrees is not enough. Surprise! And she:

Sine is 30 degrees

I hope, the first part of task I decided and I succeeded to explain to the blondes, what the sine of thirty degrees is equal to. It is now needed to decide the second half of task, with which all academy of mathematical sciences, together taken, is unable to manage even. It is needed in the Internet to find blondes which search a sine 30 degrees. Will I try to be armed with logic of blondes and I will transfer below searching queries which blondes can enter in the searching systems at the search of answer for a question: what is a sine equal to 30 degrees? And so, searching queries, dilute my comments, in order the searching systems did not separate my creation from blondes.

Sine - it mathematicians collate the knowledge with the Internet.
Sine, cosine - the authority of mathematicians appeared, to check, as mathematicians collated the knowledge.
A sine of corner - is botanists spectacled, future of Bills Gatesis, comb the clever turnips and try to remember the school course of mathematics.
Sine of degrees - smart schoolboys pelt the question on the way, what quicker cut with this nasty thing and continue a game.
Table of sines, tangents - conscientious schoolboys and respectable brunettes scraped away all cognitions in trigonometry and try to collate them with the Internet.
A value of sine - is mathematicians, after the long wandering on sites with blondes, at last understood how it is better to formulate a searching query.
Values of cosines and sines - mathematicians remembered suddenly, that by a not sine single trigonometry lives by.
Is a sine what equal to? - and the first signs of presence of blondes in the Internet with their appealing spontaneity of intercourse, even with a computer.
Sines and cosines of corners. Table of values of sines. The sine of corner is equal - it mothers try to check up, as their children did lessons.
How to find a sine? - it is already the typical question of the confused blonde.
Sine of acute angle. Sine and cosine 30 - are mothers hardly, but already begin to understand that requires to be found in a task.
A sine of number - is poor mothers, they are even unaware that corners can be measured by radians.
A sine is alpha - mothers remembered, by what letter once at school they designated corners.
Calculator of sines - for help clever dads come helpless mothers.
How to find the sine of corner? - clever dads found the calculator of sine, it is now needed to know, how to use this thing.
Geometry is a sine, cosine. To get the table of sines. Values of sines of corners - it children unstucked from the virtual games and try to prove to the clever dads, that dads not correctly press on the buttons of calculator, because the sine of corner can not equal three jars of beer.
Calculations of sine - mathematicians-theorists try to steal job of mathematicians of the applied sciences performances.
To calculate a sine - it the mathematicians of the applied sciences steal job of the colleagues performances.
Sine of endlessness - it already physicists try to check up the calculations of mathematicians.
Being of sine - blondes try to know, where sines live.
Trigonometric sine - all discovered in surprise, that sines were not only in mathematics.

How many sine 30 - is a zero whole five tenth.

How many sin 30 is equal - so much, zero five.

How many will a sine be 30? - it is a question, certainly, interesting...

A table is sin alpha of 30 degrees - not quite table, but a sine and 30 degrees are exactly.

Numbers from which sines natural are - mathematicians, a blonde put question. Are there variants of answers? Honour of full-dress uniform is put on kitty.

Who can explain why sine 30 degrees 1/2 is equal? - well, infant prodigies, blonde put question. I specifically wrote the page "Why is the sine of 30 degrees equal to 1/2?"

How does a rectangular trapezoid look?

A rectangular trapezoid (also called right-angled trapezoid, right trapezoid) has such kind:

Rectangular trapezoid

A rectangular trapezoid always has two right angles. On a picture direct corners are marked little squares in tops A and D. Direct corners can be situated in two any contiguous tops of trapezoid.

Rectangular trapezoid

On this picture of rectangular trapezoid direct corners are located in tops B and С.

In mathematics there is determination of rectangular trapezoid, which over is brought and in Wikipedia: the rectangular is name a trapezoid at which even one corner of line. This classic determination of rectangular trapezoid, bluntly rewritten from sacred mathematical books. In Euclidean geometry a rectangular trapezoid always has two lines of corner, as two sides trapezoids, called grounds, are parallel. Segment BC, formative a direct corner with founding of АВ, will be always perpendicular to the second founding of CD. With one or three direct corners there can be only a curvilinear rectangular trapezoid, and it is quite another other class of geometrical figures already.

For blondes helped to draw kria, author of blog kria-tiv.

In an order to name a next natural number, what number is necessary to be added to the natural number?

In an order to name a next natural number, to the present natural number it is necessary to add 1 (one, unit). Every subsequent natural number anymore than previous on unit. We will consider a few mathematical examples of decision of this task.

Example 1. Let we have a natural number 3 (three). What next natural number? For the receipt of answer we will add to the number 3 (three) a number 1 (one).

3 + 1 = 4

Answer: by a natural number, following by a natural number 3 (three) there will be a number 4 (four).

Example 2. a natural number is Given 16 (sixteen). What natural number following? We add to the number 16 (three) a number 1 (one) and we will get a right answer.

16 + 1 = 17

Answer: natural number 17 (seventeen) will be following by a natural number 16 (sixteen).

Example 3. What natural number does stand after a number 18 592 (eighteen thousands five hundred ninety two) in the natural row of numbers? To the number 18 592 (eighteen thousands five hundred ninety two) we add a number 1 (one) and we get a next number from the row of natural numbers.

18 592 + 1 = 18 593

Answer: number 18 593 (eighteen thousands five hundred ninety three) costs following by a number 18 592 (eighteen thousands five hundred ninety two) in the natural row of numbers.

A general formula for being of next natural number looks so (a plus one is evened b):

a + 1 = b

where а - is the set natural number
         b - next natural number

To check up the got result for the first 120 (one hundred twenty) natural numbers it is possible on the table of natural numbers.

Integers determination

Oddly enough, looked over three different reference books on mathematics - all of them bashfully hold back determination of integers. There was determination of great number of integers in Wikipedia. Specially I will quote this masterpiece of simplicity : the great "number of integers is determined as shorting of great number of natural numbers of relatively arithmetic operations of addition and deduction". Does remain only to ask authors, how many years it will have to the aliens to sit in our academies, they will understand before, what we name integers?

By analogy with determination of natural numbers, we will formulate determination of integers from Nikolay Khyzhnjak: all numbers which can be got as a result of addition of positive and negative units are named integers.

We will consider examples. Number 2 (two) is an integer, as it can be got addition of two units:

1 + 1 = 2

Number -2 (minus two) is an integer, because he can be got by addition of two negative units:

(-1) + (-1) = -2

From the determination formulated by me quite logically do we get an answer for a question: "is there a zero by an integer?". Yes, a zero is this integer which can be got addition of positive and negative unit:

1 + (-1) = 0

A zero is not a positive or negative number.

Natural numbers definition

Determinations of natural numbers, which over are brought in Wikipedia and mathematical literature, contain whatever, except mathematics. If we decided to divide numbers with that it was simpler to rule above them, then competent determination of natural numbers will not prevent to enter mathematically.

Natural numbers definition from Nikolay Khyzhnjak: unit and all numbers which can be got as a result of addition of units are named natural numbers.

For those, who considers that a zero belongs to the natural numbers, this determination will sound so: zero, unit and all numbers which can be got as a result of addition of units, is named natural numbers.

What did we do? We account sticks in a hand replaced units in mathematics. Now we will check in practice, as it works. We will consider a number 2 (two):

1 + 1 = 2

Number 2 is a natural number, because it can be presented as a sum of two units, that corresponds to two account sticks or two other objects "for an account in natural way" (quotation from classic determination of natural numbers).

We will take more difficult example. If broken number 7,5 to divide into other broken number 2,5, will there be a result by a natural number?

7,5 : 2,5 = 3

Yes, as a result of division of two broken numbers we got a natural number 3, as it can be got as a result of addition of three units.

1 + 1 + 1 = 3

If a number scatters on units without noise and dust, such number is natural. For example, number 2,5 (two with a half) is not natural, because except two units with a frightful crash fractional part of number of 0,5 is pushed aside:

1 + 1 + 0,5 = 2,5

Another example. Number -4 (minus four) is not natural, as at decomposition on units a sign falls off minus and lifts the whole heap of dust. Negative numbers it is impossible to get addition of positive units. By the way, in the dust of negative numbers of mathematics roamed, as hedgehogs are in fog. Instead of that to understand reasons of dustborne storm, they thought of the module of number, than yet more all tangled.

I hope, my determination will help you it is better to be oriented in such different names of such identical numbers.

Does a broken number can to be natural? - no, broken numbers do not behave to the natural numbers.

Natural numbers are determination

Determination of natural numbers from Wikipedia: natural numbers are numbers which arise up in natural way at an account. And farther already fuss went with different approaches at by determinations of natural numbers. I have the special page with the generally accepted look to the natural numbers.

I can give another determination of natural numbers: natural numbers are numbers, used for the account of objects or for pointing of sequence number of one or another object among homogeneous objects.

In order to avoid problems in the process of studies, urgently I recommend to learn that determination of natural numbers, which is given in your textbook. In case of necessity, will pronounce him, as quotation from Mathematical Scripture. Looking at natural numbers is possible in the table of natural numbers. If want though something independent to consider, I recommend to read my determination of natural numbers.

Now a bit about history of natural numbers. It appeared in mathematics in those ancient times, when all blondes were natural. As follows from determination of natural numbers, natural numbers are those numbers which it is possible to count blondes. Exactly in honour the natural blondes of mathematics named numbers "natural". If it is possible to count blondes some number - this number natural means, if to count blondes it is impossible - a number means is not natural.

Mathematics for blondes

For example. Shot the one second is not a natural number. Where did you see half-blondes? Or one and a half blondes? All broken numbers are not natural numbers. Negative numbers similarly do not behave to the natural numbers. It is possible to count negative numbers, how many blondes do not seize to every concrete mathematician, but these blondes will be not natural, and virtual. What numbers are natural? Positive integers are natural.

Now interesting question: is a zero a natural number? In the different sacred books of mathematicians there are different opinions on that score. One add a zero on the face of sacred natural numbers, other refuse to the zero in such honour. Most correct decision of this problem - read, that it is written in a textbook or straight will ask the teacher. It is necessary to acknowledge that with a question adding of zero on natural numbers little disorder is created in mathematics. And this little disorder sometimes grows into large mathematical chaos, when business comes to the zero. Division by zero - one of examples.

Table of natural numbers

Table of natural numbers from 1 (one) to 200 (two hundred) is a sequence of positive integers which in mathematics it is accepted to name natural numbers. Here you can get the table of natural numbers free of charge. In a table the natural row of numbers is presented from 1 (units) to 200 (two hundred).

Attention! Zero is a natural number! In Russian mathematics a zero is not a natural number.

Table of natural numbers

The sequence of natural numbers, formative the natural row of numbers is evidently presented in a table. Can this table bravely be named Periodic System of Natural Numbers from Nikolay khyzhnjak))) Why is my row of natural numbers closed on a number 120? Simply to me, as to any respecting itself cat, it was to write laziness farther. If you need the table of natural numbers of largenesses (well, there, to cover a settee) vitally, write in the comments ordering from sizes - I will execute certainly. To the first pussy-cat - free of charge)))

The table of natural numbers will help you masterly to determine the amount of different numbers in natural numbers and satisfy other mathematical needs. The table of natural numbers is worked out in the secret laboratories of extraterrestrial civilizations specially for blondes, in case of cruel interrogations inquisitors from mathematics))) are Presently examine the question of creation of table of natural numbers for a flush-off on mobile telephones (such to itself mathematical body armour).

To get the table of figure (numerals) from 1 to 20 - the table of natural numbers is here presented only, but not figure (numerals). Figure (numerals) exists only ten.

Natural figure (numerals) - numerals are Arabic, Roman et al, and natural are numbers.

Figure (numerals) 100 - one hundred is a number and this number 100 consists of three figure (numerals) is one unit 1 and two zeros 0 and 0. All together these three figure (numerals) form a number 100 - one hundred.

Draw a table of values for the first 6 natural numbers.

Table of primes 2 to 20,000.

Trigonometric table

A trigonometric table is a table of values of trigonometric functions. This trigonometric table contains corners in degrees and radians, that very comfortably for translation of degrees in radians and vice versa, radians in degrees. The table of trigonometric values of functions is made with roots square and by shots, that allows to abbreviate shots at the decision of school examples. The sine of sin, cosine of cos, tangent of tg, cotangent of ctg, secant of sec, cosecant of cosec, is presented in a table.

Table of values of trigonometric functions

To facilitate life of blondes, we yet not once will take apart this trigonometric table on the lines of sines, cosines, tangents, cotangents, secants and cosecants, on the columns of degrees and radian, on the separate squares of values of trigonometric functions.

In a trigonometric table presented sine of corner of sin 0, 30, 45, 60, 90, 180, 270 and 360 degrees or 0, pi/6, pi/4, pi/3, pi/2, pi, 3pi/2, 2pi radian. Values of sine of corner of sin 0, 1/2, a root from 2 is divided by 2, a root from 3 is divided by 2, unit and minus unit. A line opposite the letters of sin is named yet table of sines.

The table of values of trigonometric functions contains the cosine of corner of cos 0, 30, 45, 60, 90, 180, 270, 360 degrees. If to transfer these corners in radians, we will get 0 pi, pi/6, pi/4, pi/3, pi/2, pi, 3pi/2, 2pi radian. A table of cosines of these corners is a line opposite the letters of cos, in which unit, root, is writtenin from three divided by two, a root from two is divided by two, one second, zero and minus unit.

First two lines of this table of sin and cos is the table of sines and cosines.

The table of tangents was hidden below than table of sines and cosines in a line with two letters of tg. Oddly enough, but the same are here present tangent of corner of tg 0, 30, 45, 60, 90, 180, 270, 360 degrees. And in radians they are transferred just 0 pi, pi / 6, pi / 4, pi / 3, pi / 2, pi, 3 pi / 2, 2 pi radian. The values of tangents of these corners make a zero, unit is divided by a root from three, unit, root from three and hyphen which is sometimes replaced by the sign of endlessness. It means that mathematicians can not define the value of trigonometric function tangent for corners 90 and 270 degrees. So, blondes, not despair, even mathematicians can not all!

Yet below there is a table of cotangents. We will repeat once again those corners for which the cotangent of ctg is writtenin in a trigonometric table : 0, 30, 45, 60, 90, 180, 270, 360 degrees. And once again we will be trained to transfer degrees in radians: 0 pi, pi/ 6, pi/ 4, pi/ 3, pi/ 2, pi, 3pi/ 2, 2pi radian. The cotangent of corner of ctg begins from a vagueness, marked a hyphen, along go root from three, unit, unit is divided by a root from three, zero.

Two middle lines of trigonometric table are folded in the table of tangents and cotangents.

Two last lines of trigonometric table occupy a secant which is designated sec, and cosecant which is designated cosec. As these trigonometric functions are reverse to the cosine and sine, the values of these functions are reverse to the values of cosine and sine. I turn your special attention on that mathematicians once again made an effort tangle blondes, violating logic of application of prefix of Co. It turned out for them, that a secant is a trigonometric function, reverse to Cosine, and Cosecant, csc - reverse to the sine. Naturally, that for a secant and cosecant there are corners, the values of functions for which are not certain.

Trigonometric  table

Large trigonometric table with fractions and square roots.

Trigonometric table 0 - 90 degrees

You might be interested in:

Trigonometric circle sine cosine
Trigonometric table of sines and cosines
Trigonometric table tangent cotangent in degrees
Trigonometric table in radians
Why is the sine of 30 degrees equal to half one?
Legend about Sine and Cosine

How to find a value:

Table of values of the trigonometric functions
cosecent trigonometric tablestable 4pi/3-2pi
table of trigonometric functions 30 to 360 degrees for students
radians degrees sine cosine tangent chart
six trigonometric functions
mathematics sin cos value table - WAW! it can be seen here!

cos from 0 to pi
table of 6 trig functions 0 to 360
sin 90
sin cos 0 30 45 90 180
trig table pi over
seperate sin cos
table tangent radians pi - you can find on this page.

Trigonometric function of basic corners table - the most widespread in textbooks and examples corners over are brought in a trigonometric table.

Table cosine sine tangent and cotangent free of charge - all these functions are here collected in one table and looking at them is possible quite free of charge.

Trigonometric table of sin cos tan cot - and yet here is sec and csc, in degrees and radians.

Tangent of pi on 4 - how mathematicians are not perverted only, to mask usual unit.

Sine 180 degrees equal - and so mathematicians can mask an usual zero. Straight not mathematics, and woman bag some - while anything will find in her...

sin zero - well here, another zero they hid, true not very much far, but insidiously - in cos 0 after a zero unit is hidden. Try to be not here tangled.

A table of sines and cosines is in radians - well, here the not greatest table, but some radians are present. It will be needed to create anything monumental.

Table of often meeting values of sine of cosine of tangent of cotangent - here are necessary to you values. If you consider that other values meet more often, will report to me about it, I will correct the annoying misunderstanding.

cot 225 degrees - minus is equal to unit (- 1). In a table for the botanists of it it is not, had to hit upon. Bad trigonometric table, it is needed to draw other, specially for series "Trigonometry for blondes".

A table of sines and cosines is in fraction - yes, exactly as a fraction the values of six trigonometric functions are writtenin for some corners in a table on a picture.

School table of tangent - I am here and tangent as a shot, specially for schoolchildren.

To calculate cosecant - here are cosecants in a table.

Table of tangents with the use of pi - after absence the best, while I can offer this table only.

sin cos tg column row - yes, here in a trig table there are both rows and columns.

Value of 30 degree sin table fraction - yes, yes, yes it here.

cosine, tabel trigonometric, tabelul trigonometric - welcome on this page.