1) x + 2 = x
2) √x = -1
3) x/0 = x
Probably, on mathematicians these unsolvable equations operate, as a boa on rabbit and infuse with in their souls the awesome trembling. I am deprived such superstitions. Moreover, the author of this idea, Vag, tossed up to me a magic stick:
"Decisions does not have" means that it is WELL-PROVEN that such circumstances at that problem abode by ARE not.
Today we will consider circumstances at that terms at least one of equations observed. Consequently, at least one unsolvable equation we will decide now.
But to begin would I like not from a decision, and from an answer to the question: "Where these unsolvable equations appeared from?" Me it seems to, here from where...
...A mother bought to the child a new toy are blocks with an alphabet. She collected a few words from blocks, read them and gave blocks to the child. A mother took up the everyday businesses.

A child with enthusiasm began to fold blocks. Finishing to lay out series from the blocks taken by chance, he put question to the mother:
- That is here written?
- Nothing. You laid down letters wrong, - a mother explained, - When will learn letters, then you will be able correctly to fold blocks.
A child was very offended and burst into tears. What a more mother tried to quiet him, the he cried stronger. Then a mother said:
- Well, try once again, I necessarily will read you.
Bustling a nose, a child at random took a few blocks and laid down them in series.
- What did I write? - he asked with tears.
Farther not to disorder a child, a mother answered:
- In mathematics a certain integral is so designated in limits from Old Testament to nine evening, taken on the surface of asynchronous point.
A child quieted down puzzled. He understood nothing, but something disturbed him in this phrase. A bit thinking, he asked:
- And will a fairy-tale be at nine o'clock of evening?
- It will be necessarily. Where will she go? - a mother answered very confidently.
Very attentively looking to the mother in eyes, a child by touch took a few blocks and laid down another abracadabra.
- And it what did I write? - he asked with distrust, not tearing away a look from mother eyes.
If a mother will not look at blocks will say that is written there, she means cheats him. If will look - she means that is written there really reads.
A mother looked another portion of incoherent words at blocks and crfpfkf. A child was happy. He has the most clever mother! Farther this game proceeded until child not tired of. He really had a clever mother that knew many clever words.
A child grew up then, learned letters, began independently to fold words from blocks. When he became adult, he forgot playing blocks, but faith that for him the most clever mother, remained...
Just as a child made blocks, mathematicians worked out unsolvable equations from mathematical symbols. A child grew and became adult, mathematicians so remained in pampers of the determinations.
Now we will pass to business.
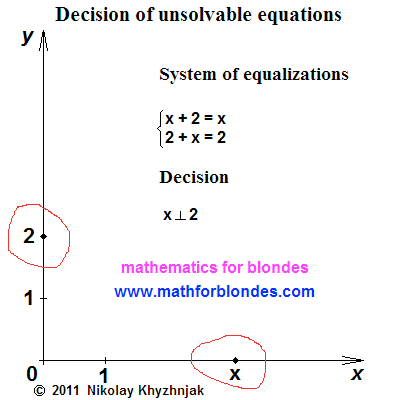
Decision of the first unsolvable equation
x + 2 = x
This equation traditionally can be taken to next equality
2 = 0
As this equality did not look wildly, but in mathematics such quite possible. I will say anymore, the first equation is only one equation from the system of two equations, that has one common decision. The second equation looks so:
2 + х = 2
This equation is usually taken to equality
х = 0
The decision of this system of equations looks so:
х ⊥ 2
It means that the numbers taken by you are on perpendicular numerical axes, therefore the rules of ordinary arithmetic give such result.
I especially want to underline that all the known rule "from transposition of elements a sum does not change" in this case stops to work. A result depends on that, what number you take for basis at implementation of action of addition.
The further decision of this system of equalizations is possible two methods resulting in different results.
First method. Turn of one of numerical axes on 90 degrees and passing to the rules of ordinary addition. Undeniable equality will ensue:
x + 2 = x + 2
In this case the laws of symmetry of mathematical actions begin to work.
Second method. Remaining in the rectangular system of coordinates, to apply the methods of vectorial algebra and find a sum on the theorem of Pythagoras, where х and 2 are the cathetuses of rectangular triangle, and result of addition - by a hypotenuse. If you consider that application of facilities of vectorial algebra is impossible, when on the ends of sticks the tips of pointers are absent, then it is your personal problems already. In this case both equalizations of the system are taken to the identical decision:
x + 2 = √(x² + 2²)
It is done away with the decision of this unsolvable equalization. you need to be only determined with the desires - what result needs you.
Decision of the second unsolvable equalization.
√x = -1
This decision caused most difficulties from the simplicity. We erect both parts of equalization in a square and get an answer:
x = 1
This equalization is taken to equality:
+1 = -1
In the parallel instances of mathematics usually begin to dissert upon the modules of numbers. I will say that before to put signs before numbers, it is needed to understand sense of positive and negative numbers.
Decision of the third unsolvable equalization
x/0 = x
The decision of this equalization is taken to equality:
1 = 0
Personally for me there is nothing unusual in this equality. This one of basic equalities of mathematics, without that mathematics in principle is impossible. If our mathematicians until now do without this equality, then only due to the pampers. At exposition of mathematics on the pages of this web-site I will repeatedly call to this equality.
To accept the solutions offered by me or not accept is this your personal file. Monkeys too nobody compelled to go down on earth. Many of them until now on branches bruise along and fully happy without pampers))))















No comments:
Post a Comment