Annotation
Number of terms
When performing mathematical operations with infinite series, for each example it is necessary to use the same number of terms in the visible part of the series. If you do not follow this rule, it can lead to an erroneous result. In mathematics, it is not customary to use any number with different rounding accuracy in one example.
Shifting series
Consider an example. If you subtract the same series without a shift from any series, the result will be zero. If the subtraction uses a shift and does not take into account the compensating group of terms, then the result will be different from zero. Suppose we have a series S. Let's write the series -S and add these two series. The result should be zero. By performing addition without shift, we get the correct result.
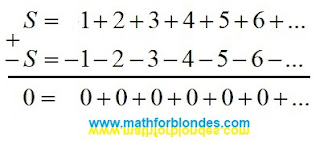 |
| Addition of infinite series without shift |
Shift by one position leads to wrong result.
 |
| Shift by one position |
A two-position shift leads to another incorrect result.
 |
| A two-position shift |
Shifts by an arbitrary number of positions allow you to get an infinite number of incorrect results.
Compliance with the rule about the same number of terms of an infinite series in one example and the use of the compensating group of terms (highlighted in curly brackets) avoids an error. But in the examples given, this method is more laborious than no shift.
 |
| The use of a compensating group of terms |
You can understand the reason for the occurrence of incorrect results if you consider the shift in arithmetic.
Let us analyze some of the most well-known examples of determining the sums of infinite divergent series.
Sum of Grandi's series
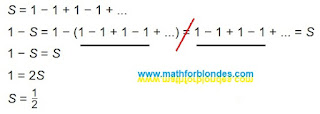 |
| Sum of Grandi's series |
The second line contains an equals sign between two different sums: one sum consists of five terms that are not equal to zero, the second consists of four. In this example, the optical illusion of the equality of different sums is used, if one term is hidden behind the three-dots of infinity.
The sum of the alternating series
 |
| The sum of the alternating series |
In the proposed solution, the same series is represented by a different number of terms: from four to six. When performing mathematical operations and rearranging terms, the compensating group of terms is ignored. Two other methods for obtaining the sum 4s are not considered: addition of series without shift and multiplication of the original series s by 4. Both these methods give the same result, which indicates the mathematical accuracy of the calculations performed.
 |
| The sum 4s |
Sum of natural numbers
 |
| Sum of natural numbers |
Typical errors of these calculations are shown in the example above. Mathematicians did not check the solution: the original series c, multiplied by minus three, is equal to:
 |
| Solution verification |
Conclusion
More interesting math ideas on the page "My Math"
























